Barry Mazur is the Gerhard Gade University Professor in the Department of Mathematics at Harvard University.  Barry is one the world’s leading experts on number theory, and his work focuses on Diophantine geometry and elliptic curves. His research in topology is also legendary, and he settled the generalized Schoenflies problem as a doctoral student.

He is highly awarded, having received the Steele prize, Cole Prize, Chauvenet Prize, and Veblen prize. In 2011, he received the National Medal of Science from President Obama. Barry is also a fellow of the American Mathematical Society and the National Academy of Sciences.

I met Barry in 2004 while visiting Fan Chung Graham in San Diego. He was attending a conference in honor of Persi Diaconis and Fan introduced us. I was initially intimidated meeting such a mathematical giant, but I recall him greeting me with a warm smile and politely shaking my hand.

In the interview, he spoke thoughtfully and deeply, and he has an infectious laugh that immediately put me at ease.

Enjoy!

***

AB: Did you show an interest in mathematics as a child or did that come later?

BM: I remember being puzzled when I was a very small child, by patterns that have what might be called a ‘mathematical feel.’

Here is an example, which I have written about. I was fascinated by this simple question: if you count the fingers on one hand, you have five, and if you count the crevices in that hand, then you have four. Whether that is mathematics, I don’t know—it’s a kind of elementary thinking about patterns that I’m sure everyone does. We look at a flower, or a teacup, and we’re struck by its symmetry or its engaging lack of symmetry—by any structure that could be thought of as geometric.

AB: Was there a family member or teacher, prior to your university education, who supported you to study mathematics?

BM: My father would always test me with little puzzles. I don’t know how old I was, but I was appropriately young for this type of puzzle: what number when you double it and add one do you get eleven? My approach at that point was brutal trial-and-error. Frustrated, perhaps, by my experimental approach, at one point my father said, “I will show you a secret.” He wrote at the top of a blank sheet of paper ‘Let x be the number when you double it and add one you get eleven.’ Then he carefully wrote out the rudiments of doubling x and adding 1, and then suitably unveiling the x to find that it is equal to 5.   He was very fastidious about his instructions.   I was both beguiled and happy with that. I cherished its secrecy as much as its effectiveness.

He would quiz me from time-to-time and I would find answers for him, armed with our family secret. I was astounded, some years later, to find this very family secret being revealed on the blackboard to the entire math class by a teacher. Of course, it is rather its un-secrecy, the availability of mathematics to everyone, that we should press for!

AB: You completed your doctorate at Princeton in the 1950’s. Would you tell us about the Mathematics department at Princeton back then? How did you end up working with your supervisors there?

BM: Princeton was wonderful. At that time, it was vibrant with algebraic and differential topology. John Milnor and Norman Steenrod were there, as well as a number of other great innovators of various aspects of topology.

But more important to me than Princeton was M.I.T. where I did my undergraduate studies. I arrived at M.I.T. with a passion for electronics. What enthralled me about the subject is what one might call ‘the philosophical aspects of electronics having to do with action at a distance’ and the issue of electromagnetism. I was deeply impressed by the amateur radio enthusiasts who I had met in my high school years. I thought that ‘radio waves,’ whatever they were, constituted a great mystery that I had to get to the bottom of: how is it that energy leaving the antenna of a transmitter somehow manages to find the antenna of a radio receiver? What is it doing between the moment it leaves and the moment it arrives? This was my motivation for going to M.I.T. and trying to learn the mathematics I needed to understand this.

The first day I got to M.I.T., I discovered the library and the immense resource of books and journals, all about the very mysteries I wanted to understand. What I quickly realized, though, was that it was precisely the mathematics behind those mysteries that I was really interested in. I immediately changed my emphasis from electronics, to physics, and then to mathematics.

I was at M.I.T. for two years and managed to get into the graduate school at Princeton in my third year. In graduate school there were, as I mentioned, wonderful teachers but not too many classes at the levels I was capable of absorbing (or, in fact, at any level). At that time, there were fewer classes than there are here at Harvard. I, like many other graduate students, was therefore left to my own resources.  I remember going to an undergraduate course in Galois Theory given by Emil Artin, and a graduate course in C*-algebras by Kaplansky (who was visiting Princeton at the time).  We graduate students would go to a course or two, but otherwise we would set up our own seminars to learn things. We cobbled together a hit-or-miss type of curriculum that probably wouldn’t have been approved by the older generation. We studied some physics, books by Arthur Eddington, lots of point-set topology, and random topics. All of it was thrown together and actually very exciting.

I was there at Princeton for a year as a graduate student. One year is not that long a time, but still, the next year I wanted a break, so I went to Paris with a girlfriend from high school. There in Paris I had the urge to try to prove the Poincaré conjecture. Paris was extremely lively back then mathematically (as it always is), and I went to a number of courses there, studying more algebra than I had previously done.

Of course I didn’t manage to prove the Poincaré conjecture, but I did prove something that I called Lemma 1, a lemma that I didn’t think of as a significant step towards the Poincaré conjecture. I came back to Princeton at the end of the first year, forgetting about my Lemma 1.  But one day, in the common room of Princeton I heard Ralph Fox, the great knot theorist, talking about various interesting open problems in topology. He mentioned something he called the Schoenflies problem and I immediately recognized it as my Lemma 1. So I brashly told him, “I can do that!” His response, as would only be natural, was quite dismissive: he said that if I could do that he would make me a professor at the Institute.

I wrote up my proof and showed it to Christos Papakyriakopoulos. He was a long-term visitor at Princeton and a great topologist. As I started to explain my proof on the backboard of his (tiny) office, he said: “prove it in dimension 4.”  But Lemma 1 works in every dimension, so I told him I could—without changing the words of my proof—prove this Schoenflies problem in any dimension n. He insisted, though, that I do it in dimension 4.  In my explanation of my proof to him, every so often I would forget and say “n” rather than “4” and he would correct me: “we are in dimension 4” he’d say. Later, this turned into something of a running joke between us. He was gracious and supportive, but the number 4 plays no role in my proof.

Another great topologist, R.H. Bing, was at Princeton at the time, visiting the Institute For Advanced Study. I showed my proof to him and he, too, was incredibly gracious. He said I should give a lecture about this at the Institute, and explained to me…among other things… how to actually give a lecture, and how to draw the diagrams I had to draw. Bing’s mathematical work is magical and it delves so deeply into the essence of three-dimensional topology. A striking example of this magic is his amazing theorem that—describing it perhaps a bit too succinctly—the double of the wild component of the Alexander horned sphere (that is, two copies of this component sewed together on their boundary) is homeomorphic to the three-dimensional sphere.

I brought the written version of my proof of Lemma 1 to Ralph Fox and I said I wanted this to be my thesis. He didn’t say much, but I did graduate and received an invitation from the Institute for Advanced Studies to study for a year as a post-doc (I assume that it was thanks to him).

J. Robert Oppenheimer was the director of the Institute at the time and very generous with his time. He once drove me to the house of J.W. Alexander (the creator of the Alexander horned sphere) who was retired from the Institute but lived nearby. I vaguely remember that we did have a conversation, but I was quite tongue-tied.

AB: While your early work focused on topology, later you moved to work in number theory, especially Diophantine geometry and elliptic curves. Can you explain for the layperson how geometric or topological thinking influences the study of numbers?

BM: It’s always shocking when you see two intuitions that seem to have nothing to do with each other somehow combine and reinforce one another—then synthesize to form some new powerful viewpoint. The names of some fields of mathematics already suggest this: a name with a noun and adjective combined like algebraic geometry gives you a hint there is some synthesis built from the combination of algebra and geometry. That happens more than one would imagine when you study mathematics.

One of the great links between topology and number theory is between knots (which are closed strings in three-dimensional space) and prime numbers. It would be difficult to explain what the analogy is without being technical; so I won’t. But for me, that was a helpful springboard to move from topology to number theory—a way of passing from a topological intuition and merging it with an arithmetic intuition about primes. That is one link and there are many others.

I was also interested in dynamical systems. There are many types of dynamical systems, but the type I was thinking about are `discrete dynamical systems.’ Such a dynamical system is based on a transformation T of a geometric space to itself. What happens when you perform that transformation again and again:  for example, take a point x and see where it is sent to after iterations of that transformation—that is, T(x), T(T(x)), T(T(T(x))), …   You get an orbit.  Such orbits are often fascinating; they often have some interesting topology, there may be attracting or repelling orbits, they may be densely distributed. Dynamical systems are very beautiful in their own right, but they also have incredible utility and application in disciplines such as physics and other subjects. It occurred to me and Michael Artin to apply algebraic geometry to a question in dynamical systems. For this, I had to learn some  “real algebraic geometry” (the theory of Nash manifolds) and that got me hooked, and I became more interested in algebraic geometry and eventually, number theory.

AB: What research topics are you working on most recently? You can be more technical here, if you like.

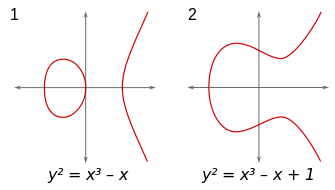
BM: I am interested in rational points, which are solutions of polynomial equations—the coordinates of these points being rational numbers (or fractions). Take an equation such as y² = x³+1 which you can visualize as a curve in the plane.  The problem is to find the full list of points in the plane that have rational coordinates and happen to lie on that curve.  This type of problem has an extraordinary beauty, but is also often important for applications or other mathematical pursuits.

I mentioned y² = x³+1 as my example because among polynomial equations in two variables, linear and quadratic polynomials have one type of behavior, high degree polynomials quite another, and there is a very curious interface—between these two different ‘types of behavior’ that occurs with polynomials of degree 3:  you are in the land of elliptic curves. Elliptic curves are seemingly confined and specific, but they are ubiquitous in mathematics and show up in as many theories you can imagine such as complex analysis, number theory, lattices, and even in the study of heat equation.  In finite fields, elliptic curves become the foundation of coding theory and other aspects of the practical world. I’m told that every time you use your bank card this involves elliptic curves in the manner in which information is encoded. The basic issues in pure arithmetic and number theory depends on understanding elliptic curves.

AB: Your latest book Prime Numbers and the Riemann Hypothesis present these topics to a math undergraduate.

BM: Actually, an engaged high school student can read the book. It’s not a passive read as we are inviting people to do our computations or do them in different style. I was also thinking that engineers could well be interested in it.

AB: I’ve tried to explain the Riemann hypothesis to non-mathematicians with mixed success. How you would explain the Riemann hypothesis to someone who has a limited math background?

BM: There are steps in my expository game for this. The first is to make sure people understand the importance of primes. If you don’t realize the primes are interesting, then the Riemann hypothesis is not interesting. The second thing to realize is that prime numbers have on the one hand such erratic behavior: 2, 3, 5, 7, 11, 13, 17, 19, … They don’t seem to have a clear mnemonic that will allow you to remember the first 100 primes, say, like there would be for the first 100 numbers divisible by 10.  The primes seem to come randomly and they keep coming (that is, there are infinitely many primes).

But then—and it is quite impressive—when you look at them from afar they seem to have such clear structure. Draw a graph (on one standard-size page) that charts the number of primes less than x, and where the range is for values of x between 1 and, say, 40, and it looks like the most randomly constructed staircase. And now draw one where the range is for values of x between 1 and, say, 100,000 primes and you get a strikingly smooth graph! Looking at primes from afar, their erratic turbulence disappears. That is the perplexity: from afar the graph of primes is so smooth, while from near it looks disorganized and complicated. The Riemann hypothesis continues the grand project begun by Gauss of explaining exactly how smooth that progression of primes is.

Another point is that the Riemann hypothesis is a type of conjecture that suffuses. It’s not just nice to know the answer—but once we do know the answer we also know much more: so many other pieces of mathematics depend on knowing the validity of the Riemann hypothesis.

AB: You’ve had many students over the years such as Jordan Ellenberg who’ve I’ve interviewed here. What is your advice for young people (say graduate students) studying mathematics?

BM: The first thing I hope people learn (this applies to both undergraduates and graduates) is to respect their native curiosity. When you are curious about something, then you can ask questions that matter to you, and that’s extremely important. You should follow those questions. There is an art of asking questions in mathematics that you should cultivate. It will help you enormously.

Practice the art of understanding and respecting your own questions.

AB: I’d like to close with looking forward. What would you say are some of the major directions for mathematics in the future?

BM: Mathematics is too broad to predict. Overnight, there may be a new road. For example, I went to the Arizona Winter School, which chooses a subject (often in number theory) and has senior people giving lectures. There are usually many students, who do projects on the subject that is being taught. It’s a week-long thing. Very often the subject is one that has just opened up.

This March they covered the topic of perfectoids. I won’t tell you what it is as it is rather technical. But I knew I had better learn perfectoids, so I signed up. I didn’t give lectures; I just went to learn. This is largely around the work of Peter Scholze who is extraordinary in many ways, and has developed a marvelous school of collaborators developing perfectoids. They have produced an enormous number of results in arithmetic algebraic geometry, arithmetic, and representation theory.

The first thing that struck me was that there were between 300 to 400 graduate students learning perfectoids. It’s just as it should be, as this new development opens up an area that is extremely important. The lectures and exercise sessions were really good. It was one of the most exciting winter schools I’ve been to.

Perfectoids have been instrumental for yielding quite a few important results in the last few years. Could we have guessed this development would have occurred? I think not.

We should be very open to the future of mathematics.

Anthony Bonato